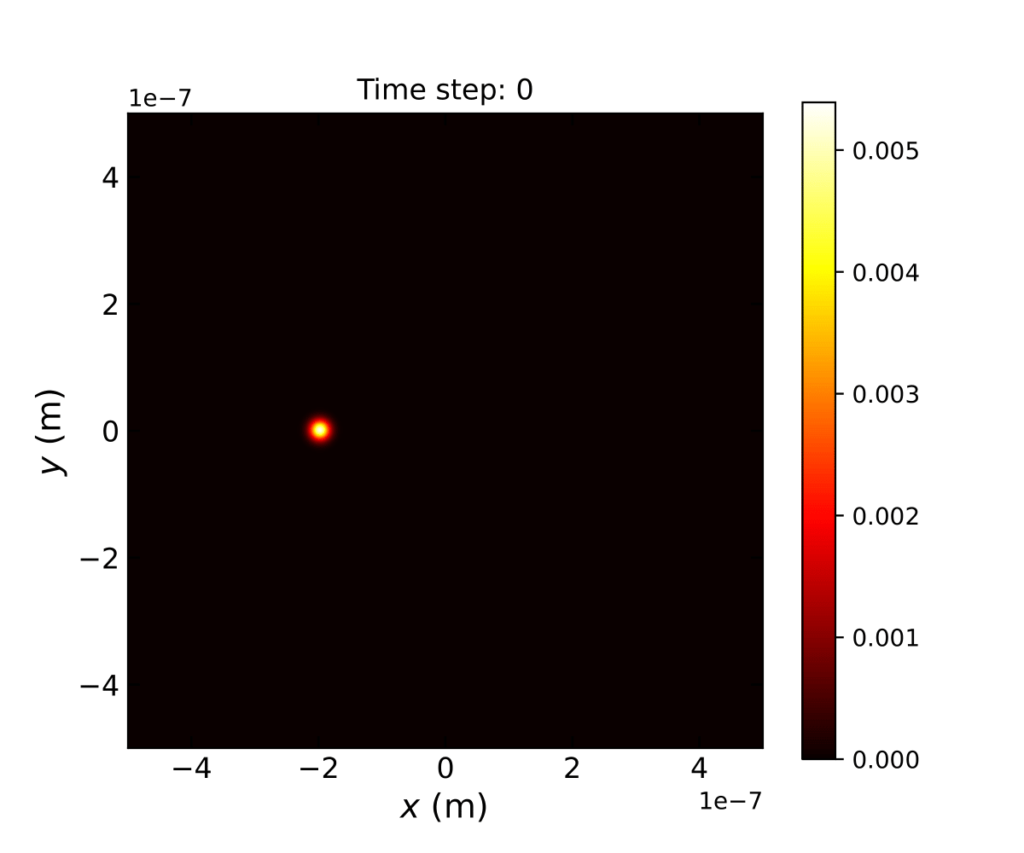
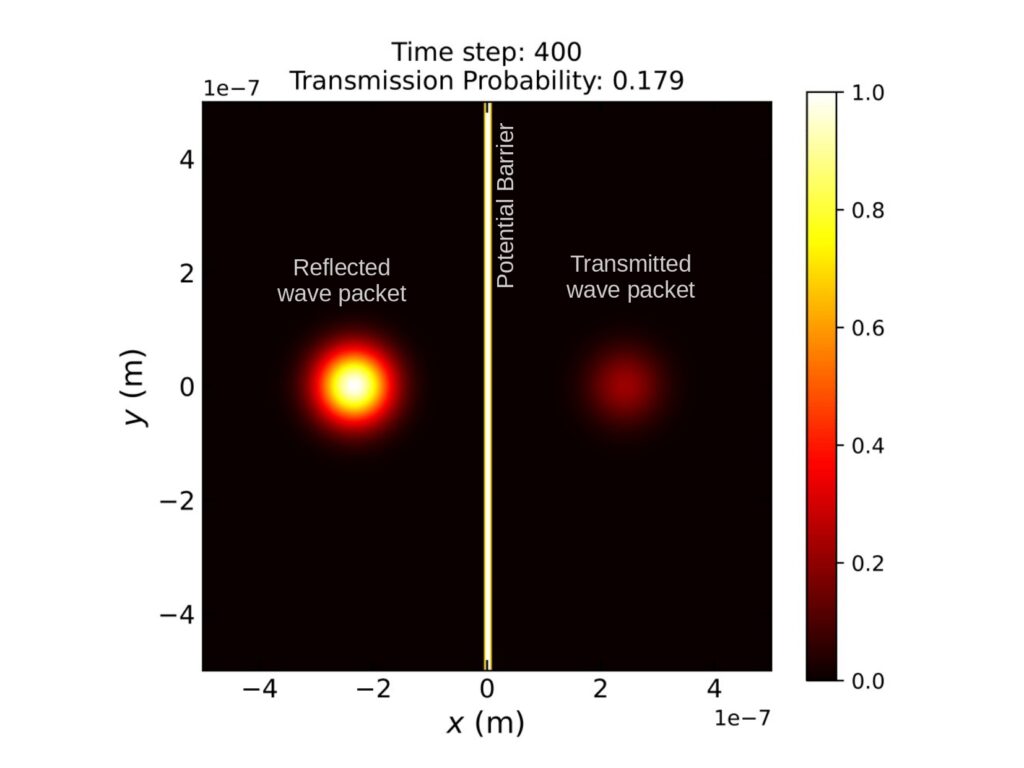
Last Updated on October 19, 2024 by Max
Derivation of Wave Functions in a 2D Harmonic Oscillator
A two-dimensional (2D) quantum harmonic oscillator is a basic model in quantum mechanics that describes a particle moving in a potential that depends on both spatial dimensions (\(x,y\)).
This model helps us understand physical systems like molecular vibrations, quantum dots, and nanoscale structures.
In this section, we will derive the wave functions for the 2D harmonic oscillator using the method of separation of variables and the properties of Hermite polynomials.
The Schrödinger Equation for a 2D Harmonic Oscillator
The Hamiltonian of a particle of mass \(m\) moving in a 2D harmonic potential is given by:
\[\hat{H} = -\frac{\hbar^2}{2m} \left( \frac{\partial^2}{\partial x^2} + \frac{\partial^2}{\partial y^2} \right) + \frac{1}{2} m \omega^2 (x^2 + y^2),\]
where, \(\hbar\) is the reduced Planck’s constant, \(\omega\) is the angular frequency of the oscillator, and \(x\) and \(y\) are the coordinates in two dimensions.
The time-independent Schrödinger equation for this system is:
\[\hat{H} \Psi(x, y) = E \Psi(x, y),\]
where \(\Psi(x, y)\) is the wave function, and \(E\) is the energy eigenvalue.
Separation of Variables
To solve this equation, we employ the method of separation of variables, assuming the solution can be written as a product of two functions, each depending on only one coordinate:
\[\Psi(x, y) = \psi_x(x) \psi_y(y).\]
Substituting this into the Schrödinger equation, we obtain:
\[\left( -\frac{\hbar^2}{2m} \frac{d^2}{dx^2} + \frac{1}{2} m \omega^2 x^2 \right) \psi_x(x) + \left( -\frac{\hbar^2}{2m} \frac{d^2}{dy^2} + \frac{1}{2} m \omega^2 y^2 \right) \psi_y(y) = E \psi_x(x) \psi_y(y).\]
Dividing by \(\psi_x(x) \psi_y(y)\) and rearranging, we get:
\[\frac{1}{\psi_x(x)} \left( -\frac{\hbar^2}{2m} \frac{d^2 \psi_x(x)}{dx^2} + \frac{1}{2} m \omega^2 x^2 \psi_x(x) \right) \\ + \frac{1}{\psi_y(y)} \left( -\frac{\hbar^2}{2m} \frac{d^2 \psi_y(y)}{dy^2} + \frac{1}{2} m \omega^2 y^2 \psi_y(y) \right) = E.\]
Since the left side is a sum of two terms, each depending only on \(x\) or \(y\), and the right side is a constant, each term must separately equal a constant. Let us denote these separation constants as \(E_x\) and \(E_y\), such that:
\[E = E_x + E_y.\]
This gives us two independent one-dimensional Schrödinger equations:
\[-\frac{\hbar^2}{2m} \frac{d^2 \psi_x(x)}{dx^2} + \frac{1}{2} m \omega^2 x^2 \psi_x(x) = E_x \psi_x(x),\]
\[-\frac{\hbar^2}{2m} \frac{d^2 \psi_y(y)}{dy^2} + \frac{1}{2} m \omega^2 y^2 \psi_y(y) = E_y \psi_y(y).\]
Solution for the One-Dimensional Harmonic Oscillator
The equations above are identical to the Schrödinger equation for a one-dimensional quantum harmonic oscillator. The solutions to these equations are well known:
\[\psi_n(x) = N_n H_n \left( \sqrt{\frac{m \omega}{\hbar}} x \right) e^{-\frac{m \omega x^2}{2 \hbar}},\]
\[\psi_m(y) = N_m H_m \left( \sqrt{\frac{m \omega}{\hbar}} y \right) e^{-\frac{m \omega y^2}{2 \hbar}},\]
where, \(H_n(z)\) and \(H_m(z)\) are the Hermite polynomials of order \(n\) and \(m\), respectively, and \(N_n = \left( \frac{m \omega}{\pi \hbar} \right)^{1/4} \frac{1}{\sqrt{2^n n!}}\) is the normalization constant.
Energy Levels
The energy eigenvalues for each dimension are:
\[E_x = \left( n + \frac{1}{2} \right) \hbar \omega, \quad E_y = \left( m + \frac{1}{2} \right) \hbar \omega,\]
where \(n, m = 0, 1, 2, \ldots\).
Thus, the total energy of the 2D harmonic oscillator is:
\[E = \left( n + m + 1 \right) \hbar \omega.\]
Wave Functions for the 2D Harmonic Oscillator
Combining the solutions for each dimension, the wave function for the 2D harmonic oscillator is given by:
\[\Psi_{n, m}(x, y) = \psi_n(x) \psi_m(y).\]
Substituting the expressions for \(\psi_n(x)\) and \(\psi_m(y)\), we get:
\[\Psi_{n, m}(x, y) = N_n N_m H_n \left( \sqrt{\frac{m \omega}{\hbar}} x \right) H_m \left( \sqrt{\frac{m \omega}{\hbar}} y \right) e^{-\frac{m \omega (x^2 + y^2)}{2 \hbar}}.\]
This expression represents the normalized wave function for a quantum particle in a 2D harmonic oscillator potential. The quantum numbers \(n\) and \(m\) determine the number of nodes in the \(x\) and \(y\) directions, respectively, and the Hermite polynomials govern the shape of the wave function in each dimension.
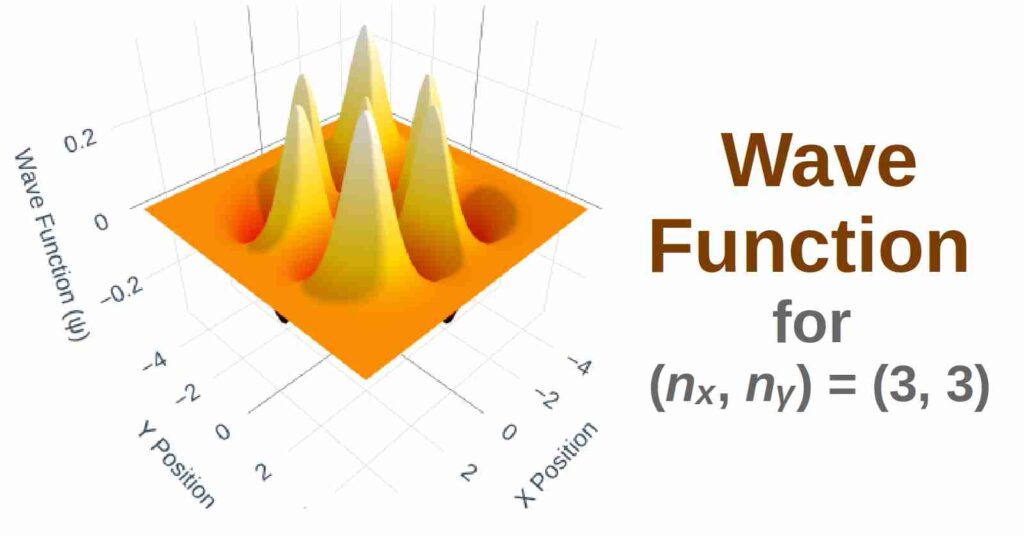
Conclusion
The 2D quantum harmonic oscillator wave function plotter offers a simple way to visualize wave functions for different quantum states as 3D surface plots. It helps users explore the spatial behavior of quantum particles in two-dimensional potentials and allows downloading images for further use.
This article also explains how the wave functions are derived using the separation of variables and Hermite polynomials, simplifying multidimensional quantum problems into one-dimensional forms.
The 2D quantum harmonic oscillator model is essential for understanding physical systems like molecular vibrations and quantum dots. It provides valuable insights into the quantum behavior of confined particles, making it a helpful resource for students and researchers in quantum mechanics.
References
[1] OpenStax, “The Quantum Harmonic Oscillator“, LibreTexts Physics.
[2] Max, “Schrödinger Equation for Matter-Wave Dynamics” MatterWaveX.Com, September 7, (2024).
[3] Weisstein, Eric W. “Hermite Polynomial.” From MathWorld–A Wolfram Web Resource. https://mathworld.wolfram.com/HermitePolynomial.html
Cite this article as:
Max, “2D Harmonic Oscillator Wave Function Plotter“, MatterWaveX.Com, September 16, (2024), URL: https://matterwavex.com/2d-harmonic-oscillator-wave-function-plotter/

I am a science enthusiast and writer, specializing in matter-wave optics and related technologies. My goal is to promote awareness and understanding of these advanced fields among students and the general public.