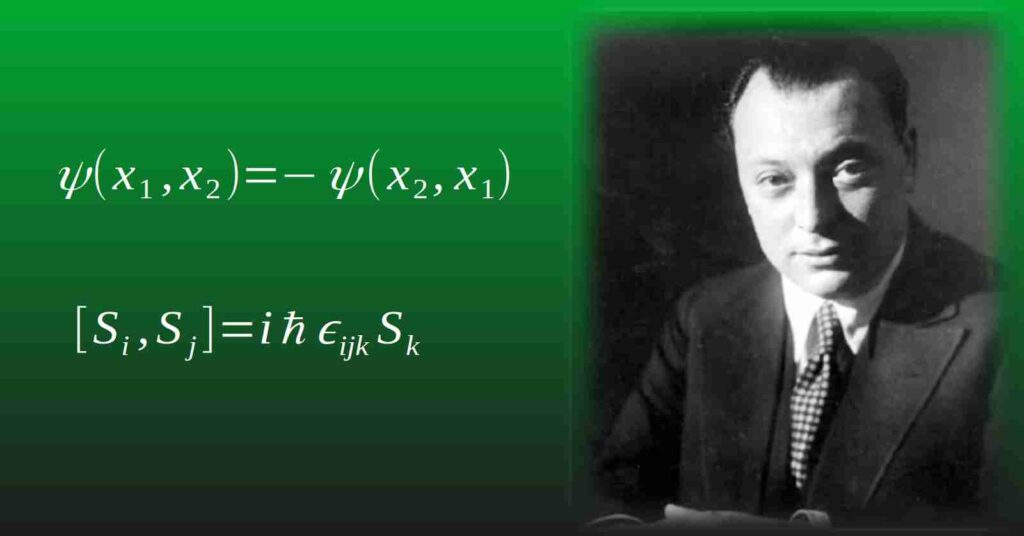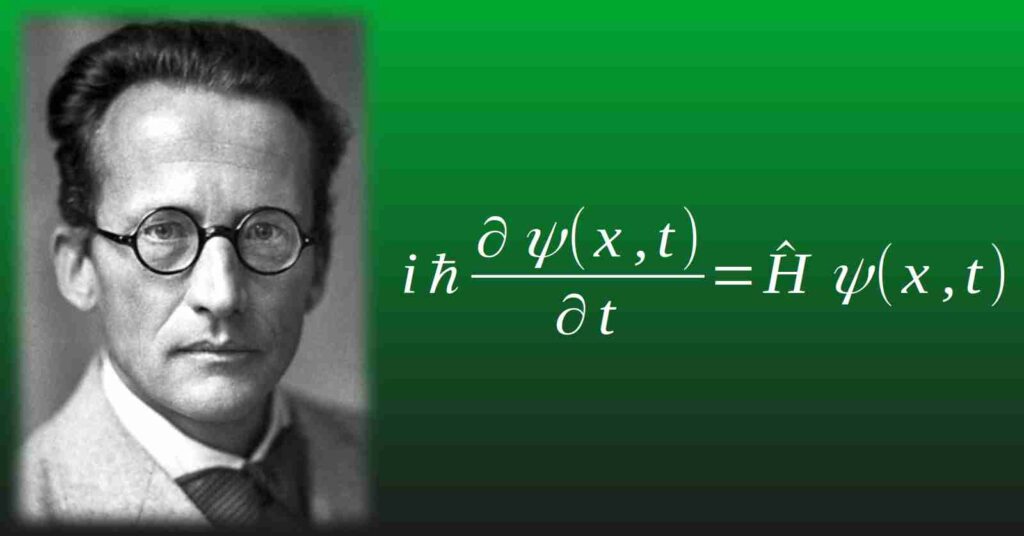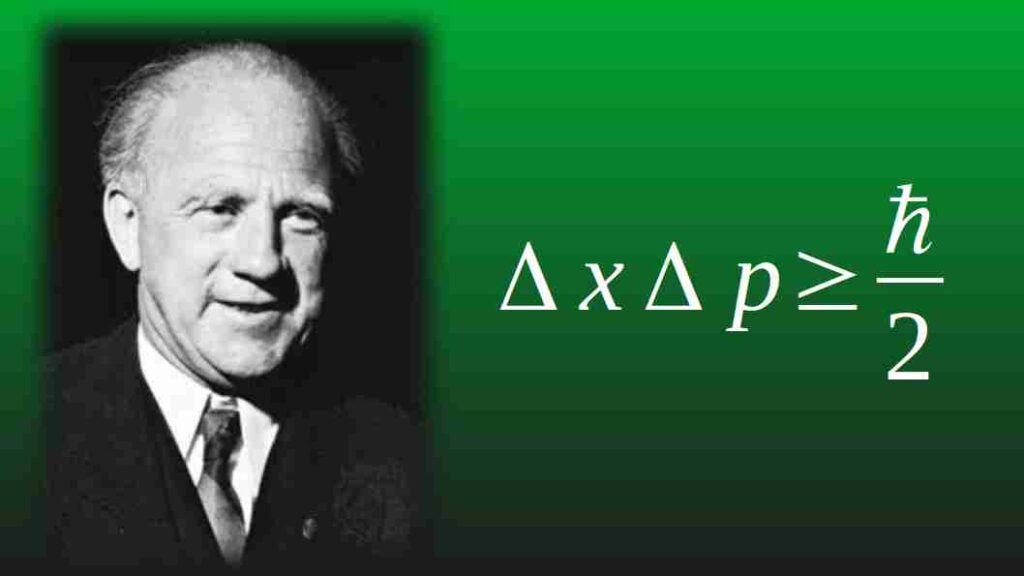Contributions of Max Born to Quantum Mechanics

Last Updated on September 14, 2024 by Max

Max Born, a German physicist and mathematician, is a central figure in the development of quantum mechanics. His pioneering work laid the foundations for understanding the probabilistic nature of quantum systems and significantly shaped contemporary quantum theory. Born’s contributions span several areas, from the formalism of wave mechanics to the interpretation of quantum phenomena.

This article explores Born’s key contributions, providing historical context, mathematical insights, and their lasting impact on modern quantum physics.

Historical Context

Max Born (1882–1970) was born in Breslau, Germany (now Wrocław, Poland). He was a contemporary of other prominent physicists, including Albert Einstein, Niels Bohr, Werner Heisenberg, and Erwin Schrödinger, during a time of rapid advancements in theoretical physics.

The early 20th century saw the emergence of quantum theory, driven by experimental findings that could not be explained by classical mechanics, such as blackbody radiation and the photoelectric effect.

Born studied under some of the most influential physicists of the time, including David Hilbert and Felix Klein, which shaped his approach to physics with a strong mathematical foundation. After the advent of quantum theory in the early 1920s, Born’s work became increasingly focused on the theoretical underpinnings of quantum mechanics.

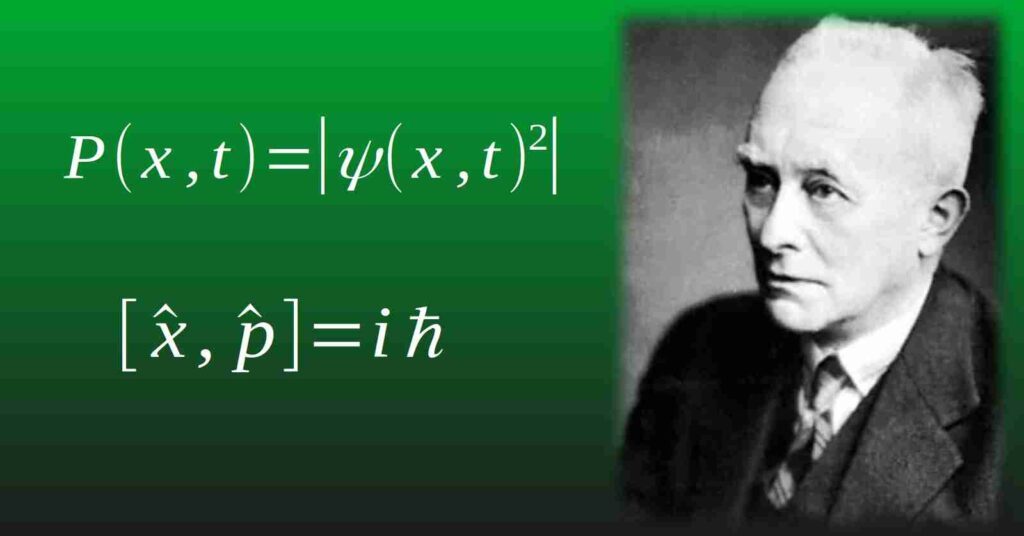
The Development of Quantum Mechanics

Born made several essential contributions to quantum mechanics, with the most notable being:

The Probabilistic Interpretation of the Wave Function

One of Born’s most significant contributions was his interpretation of the wave function, \(\psi(x,t)\), in quantum mechanics. In 1926, following the development of Schrödinger’s wave mechanics, Born proposed that the square of the absolute value of the wave function, \(|\psi(x,t)|^2\), represents the probability density of finding a particle at a given position and time.

This idea, now known as the “Born rule,” fundamentally shifted the understanding of quantum mechanics from a deterministic to a probabilistic framework.

The Born rule is mathematically expressed as,

\[P(x,t) = |\psi(x,t)|^2,\]

where \(P(x,t)\) is the probability density of finding the particle at position \(x\) at time \(t\). The total probability must integrate to 1, reflecting the certainty that the particle exists somewhere,

\[\int_{-\infty}^{\infty} |\psi(x,t)|^2 \, dx = 1.\]

The Born rule marked a departure from classical physics, which relied on deterministic predictions, and established probability as a core component of quantum theory.

This interpretation helped reconcile the wave-like and particle-like behaviors observed in quantum phenomena, such as interference and diffraction patterns.

Contributions to Matrix Mechanics

In addition to his work on wave mechanics, Born also played a critical role in the development of matrix mechanics, an alternative formulation of quantum mechanics proposed by Werner Heisenberg in 1925. Matrix mechanics treats physical quantities, such as position and momentum, as matrices that do not commute.

Born, in collaboration with his student Pascual Jordan and Heisenberg, published a paper in 1926 that developed the formal mathematical structure of matrix mechanics. Born’s deep understanding of linear algebra and matrices was instrumental in establishing this formulation’s rigorous mathematical framework.

The work of Born, Heisenberg, and Jordan laid the groundwork for understanding quantum observables’ non-commutative nature, encapsulated in Heisenberg’s uncertainty principle.

Born-Oppenheimer Approximation

In 1927, Born, in collaboration with Robert Oppenheimer, introduced the Born-Oppenheimer approximation. This method simplifies the mathematical treatment of molecular systems by separating the motion of nuclei and electrons.

The approximation is based on the fact that nuclei are much heavier and slower-moving than electrons. Thus, the electronic wave function can be solved assuming fixed nuclei positions, significantly simplifying the calculations in quantum chemistry.

Mathematically, the total wave function of a molecule \(\Psi(\mathbf{R}, \mathbf{r})\) is expressed as a product of the nuclear wave function \(\chi(\mathbf{R})\) and the electronic wave function \(\psi(\mathbf{r}; \mathbf{R})\):

\[\Psi(\mathbf{R}, \mathbf{r}) = \chi(\mathbf{R}) \, \psi(\mathbf{r}; \mathbf{R}),\]

where \(\mathbf{R}\) represents the nuclear coordinates, and \(\mathbf{r}\) represents the electronic coordinates. The Born-Oppenheimer approximation remains a cornerstone in computational chemistry, allowing the study of complex molecular dynamics and electronic structures.

Mathematical Contributions to Quantum Theory

Born’s contributions extended beyond specific theories and interpretations; he also made foundational contributions to the mathematical formalism of quantum mechanics.

Operator Theory and Commutation Relations

Born was pivotal in the early development of operator theory in quantum mechanics. He formalized the use of operators to represent physical observables, such as position and momentum.

This formalism is critical because it allows the quantization of classical quantities through the commutation relations,

\[[\hat{x}, \hat{p}] = i\hbar,\]

where \(\hat{x}\) is the position operator, \(\hat{p}\) is the momentum operator, and \(\hbar\) is the reduced Planck constant. These commutation relations underpin the uncertainty principle and the probabilistic interpretation of quantum mechanics.

Rigorous Mathematical Foundations

Born’s rigorous approach to the mathematics of quantum mechanics helped formalize the subject, bridging the gap between physics and mathematics. His work emphasized the importance of Hilbert spaces in quantum theory, where the state vectors (wave functions) reside, and operators act on these vectors to represent physical quantities. This mathematical framework remains fundamental to quantum mechanics today.

Impact on Contemporary Quantum Theory

Max Born’s contributions to quantum mechanics have profoundly impacted contemporary physics. His probabilistic interpretation of the wave function reshaped the philosophical and scientific understanding of reality, moving away from classical determinism to embrace uncertainty and probability.

Born’s work on matrix mechanics, operator theory, and the Born-Oppenheimer approximation still shapes modern quantum theory, including areas like quantum computing, quantum information science, and condensed matter physics. The probabilistic nature of quantum mechanics, introduced by Born, is also key to fields such as quantum cryptography and quantum teleportation

Moreover, Born’s emphasis on rigorous mathematical methods has inspired subsequent generations of physicists and mathematicians to explore the deep mathematical structures underlying quantum theory. His legacy is evident in the ongoing efforts to reconcile quantum mechanics with general relativity and in the pursuit of a more complete understanding of the quantum world.

Conclusion

Max Born’s contributions to quantum mechanics are foundational and transformative. His work on the probabilistic interpretation of the wave function, matrix mechanics, and the Born-Oppenheimer approximation has left an indelible mark on the field. Born’s mathematical rigor and innovative ideas have expanded our understanding of quantum mechanics and continue to influence contemporary research in quantum mechanics and its applications.