Last Updated on August 18, 2024 by Max
Quantum tunneling is a phenomenon in quantum mechanics where a particle crosses a potential barrier that it shouldn’t be able to overcome according to classical physics, due to its insufficient energy.
In classical physics, a particle lacking enough energy would be entirely reflected by the barrier. However, quantum mechanics treats particles as waves governed by the Schrödinger equation. When a particle encounters a barrier, its wavefunction doesn’t stop abruptly but instead decreases exponentially inside the barrier. If the barrier is thin enough, the wavefunction can extend beyond it, allowing the particle to appear on the other side with a certain probability. This process is what we refer to as quantum tunneling.
Historical Background
Quantum tunneling was first introduced by Friedrich Hund in 1927. While studying molecular quantum states, he observed that particles could move between different energy states by passing through potential barriers, even when classical physics suggested they couldn’t.
The theoretical framework for tunneling was further developed by George Gamow, Ronald Gurney, and Edward Condon in 1928, who independently applied the idea to explain alpha decay in nuclear physics. Gamow, using quantum mechanics, showed that alpha particles within a nucleus could tunnel through the Coulomb barrier, even when their kinetic energy was insufficient to overcome it classically.
Gurney and Condon independently arrived at the same conclusion, providing a critical understanding that alpha particles can escape the nucleus via tunneling. This work laid the groundwork for understanding quantum tunneling, establishing it as a fundamental process in quantum mechanics. It has far-reaching implications in various fields, including semiconductor physics and astrophysics.
Role of Quantum Tunneling in Various Physical Phenomena
Quantum tunneling plays a crucial role in various physical phenomena, spanning multiple domains of physics:
- Alpha decay in nuclear physics: Quantum tunneling explains how alpha particles escape atomic nuclei in radioactive decay. Despite insufficient energy to overcome the nuclear Coulomb barrier classically, these particles can tunnel through the barrier, leading to the emission of alpha particles from the nucleus.
- Nuclear fusion in stars: In stellar cores, nuclear fusion occurs at temperatures where classical physics predicts insufficient energy for nuclei to overcome their mutual Coulomb repulsion. Quantum tunneling allows protons to tunnel through this barrier, enabling fusion reactions that power stars.
- Josephson effect in superconductivity: Quantum tunneling of Cooper pairs (paired electrons) across a thin insulating barrier between two superconductors gives rise to the Josephson effect. This phenomenon is the basis for superconducting quantum interference devices (SQUIDs), which are highly sensitive magnetometers.
- Tunneling in semiconductor devices: Tunneling is essential to the function of many semiconductor devices, like tunnel diodes. In these devices, electrons tunnel through a thin barrier, resulting in negative differential resistance and distinctive electrical properties.
- Scanning tunneling microscopy (STM): STM utilizes quantum tunneling to image surfaces at the atomic level. When a sharp metal tip is brought close to a conducting surface, electrons tunnel between the tip and the surface, allowing for detailed atomic-scale imaging based on the tunneling current.
- Quantum tunneling in chemical reactions: In chemistry, tunneling enables particles like protons and electrons to pass through energy barriers in reaction pathways. This can lead to reaction rates that deviate significantly from classical predictions, especially at low temperatures.
- Quantum tunneling in biological systems: Quantum tunneling has been observed in biological systems, such as enzyme catalysis and proton transfer in DNA. These tunneling processes can affect reaction rates and contribute to the efficiency of biological mechanisms.
- Proton tunneling in DNA: Proton tunneling in DNA can lead to tautomeric shifts, potentially resulting in genetic mutations. This process influences the stability of genetic material and may play a role in biological evolution.
- Quantum tunneling in quantum computing: Tunneling is essential in the operation of qubits in quantum computers. It allows qubits to transition between states through barriers, influencing quantum gate operations and coherence times.
Theory of Quantum Tunneling Through a Barrier
In quantum tunneling, a particle can cross a barrier even if its energy is lower than the barrier’s height. This process is forbidden in classical mechanics but allowed in quantum mechanics due to the wave-like nature of particles.
Setup of the Problem
Consider a particle of mass \( m \) and total energy \( E \) approaching a potential barrier of height \( V_0 \) and width \( a \) in one dimension. The potential is defined as:
\[V(x) = \begin{cases}0 & \text{for } x < 0 \text{ (Region I)} \\V_0 & \text{for } 0 \leq x \leq a \text{ (Region II)} \\0 & \text{for } x > a \text{ (Region III)}\end{cases}\]
The time-independent Schrödinger equation for a particle in a potential is
\[-\frac{\hbar^2}{2m} \frac{d^2 \psi(x)}{dx^2} + V(x) \psi(x) = E \psi(x).\]
where \( \psi(x) \) is the wavefunction of the particle, \( \hbar \) represents the reduced Planck constant, and \( m \) denotes the mass of the particle.
The above equation can be rewritten as:
\[\frac{d^2 \psi(x)}{dx^2} + \frac{2m}{\hbar^2} \left[E – V(x)\right] \psi(x) = 0.\]
Solution in Each Region
Region I ( \( x < 0 \) ):
In this region, the potential \( V(x) = 0 \), so the Schrödinger equation becomes,
\[ \frac{d^2 \psi_I(x)}{dx^2} + k^2 \psi_I(x) = 0, \]
where \( k = \sqrt{\frac{2mE}{\hbar^2}} \).
The general solution in this region is,
\[ \psi_I(x) = A e^{ikx} + B e^{-ikx}, \]
where \( A \) and \( B \) are constants representing the amplitudes of the incident and reflected waves, respectively.
Region II ( \( 0 \leq x \leq a \) ):
In this region, the potential \( V(x) = V_0 \), so the Schrödinger equation becomes,
\[ \frac{d^2 \psi_{II}(x)}{dx^2} – \kappa^2 \psi_{II}(x) = 0, \]
where \( \kappa = \sqrt{\frac{2m(V_0 – E)}{\hbar^2}} \).
The general solution in this region is,
\[ \psi_{II}(x) = C e^{\kappa x} + D e^{-\kappa x}, \]
where \( C \) and \( D \) are constants representing the amplitudes of the growing and decaying exponential functions.
Region III ( \( x > a \) ):
In this region, the potential \( V(x) = 0 \), so the Schrödinger equation is the same as in Region I,
\[ \frac{d^2 \psi_{III}(x)}{dx^2} + k^2 \psi_{III}(x) = 0. \]
The general solution in this region is,
\[ \psi_{III}(x) = F e^{ikx} \]
where \( F \) is a constant representing the amplitude of the transmitted wave. Notably, this solution indicates the absence of a reflected wave in this region.
To calculate the explicit forms of the reflection coefficient \( R \) and the transmission coefficient \( T \), we solve the boundary condition equations for the coefficients \( B \), \( C \), \( D \), and \( F \) in terms of the incident wave amplitude \( A \).
Solving the Boundary Conditions
The wavefunction \( \psi(x) \) and its derivative \( \frac{d\psi(x)}{dx} \) must be continuous at the boundaries \( x = 0 \) and \( x = a \). This gives the following boundary conditions:
- At \( x = 0 \):
\[ \psi_I(0) = \psi_{II}(0) \quad \text{and} \quad \frac{d\psi_I}{dx}\bigg|_{x=0} = \frac{d\psi_{II}}{dx}\bigg|_{x=0} \]
Substituting the wavefunctions \(\psi_I\) and \(\psi_{II}\), we get
\[A + B = C + D\]
\[ik(A – B) = \kappa(C – D)\]
- At \( x = a \):
\[ \psi_{II}(a) = \psi_{III}(a) \quad \text{and} \quad \frac{d\psi_{II}}{dx}\bigg|_{x=a} = \frac{d\psi_{III}}{dx}\bigg|_{x=a} \]
Substituting the wavefunctions \(\psi_{II}\) and \(\psi_{III}\), we get
\[C e^{\kappa a} + D e^{-\kappa a} = F e^{ika}\]
\[\kappa(C e^{\kappa a} – D e^{-\kappa a}) = ik F e^{ika}\]
By solving these equations, we first find \( C \) and \( D \) in terms of \( A \) and \( B \).
From the first pair of equations:
\[C = \frac{1}{2} \left( (A + B) + \frac{ik}{\kappa}(A – B) \right)\]
\[D = \frac{1}{2} \left( (A + B) – \frac{ik}{\kappa}(A – B) \right)\]
Substitute these into the equations at \( x = a \):
\[\frac{1}{2} \left( (A + B) + \frac{ik}{\kappa}(A – B) \right) e^{\kappa a} + \frac{1}{2} \left( (A + B) – \frac{ik}{\kappa}(A – B) \right) e^{-\kappa a} = F e^{ika}\]
\[\frac{\kappa}{2} \left( (A + B) + \frac{ik}{\kappa}(A – B) \right) e^{\kappa a} – \frac{\kappa}{2} \left( (A + B) – \frac{ik}{\kappa}(A – B) \right) e^{-\kappa a} = ik F e^{ika}\]
These simplify to:
\[(A + B) \cosh(\kappa a) + \frac{ik}{\kappa}(A – B) \sinh(\kappa a) = F e^{ika}\]
\[(A + B) \sinh(\kappa a) + \frac{\kappa}{ik}(A – B) \cosh(\kappa a) = \frac{F \kappa}{ik} e^{ika}\]
Expressing \( R \) and \( T \) in Terms of Known Variables
To find the transmission coefficient \( T \) and reflection coefficient \( R \), we focus on the relation \( \frac{F}{A} \) and \( \frac{B}{A} \).
From the boundary conditions, we get:
\[T = \left| \frac{F}{A} \right|^2 = \frac{1}{1 + \left( \frac{\kappa^2 + k^2}{2\kappa k} \right)^2 \sinh^2(\kappa a)}\]
\[R = \left| \frac{B}{A} \right|^2 = \frac{\left( \frac{\kappa^2 – k^2}{2\kappa k} \right)^2 \sinh^2(\kappa a)}{1 + \left( \frac{\kappa^2 + k^2}{2\kappa k} \right)^2 \sinh^2(\kappa a)}\]
where \( k = \sqrt{\frac{2mE}{\hbar^2}} \), \( \kappa = \sqrt{\frac{2m(V_0 – E)}{\hbar^2}} \), and \( \sinh(\kappa a) \) is the hyperbolic sine function.
Interpretation
The transmission coefficient \( T \) indicates the probability of the particle tunneling through the barrier, while the reflection coefficient \( R \) indicates the probability of the particle being reflected.
Notably, even when \( E < V_0 \), there is a non-zero probability that the particle will tunnel through the barrier, a phenomenon that cannot be explained by classical mechanics but is fundamental in quantum mechanics. This tunneling effect is the basis for various physical phenomena and technologies as mentioned above.
Numerical Simulation of Quantum Tunneling in 2D
To solve the time-dependent Schrödinger equation (TDSE) for a 2D initial Gaussian wave packet encountering a potential barrier, and to compute the reflection and tunneling probabilities, we need to proceed with the following steps:
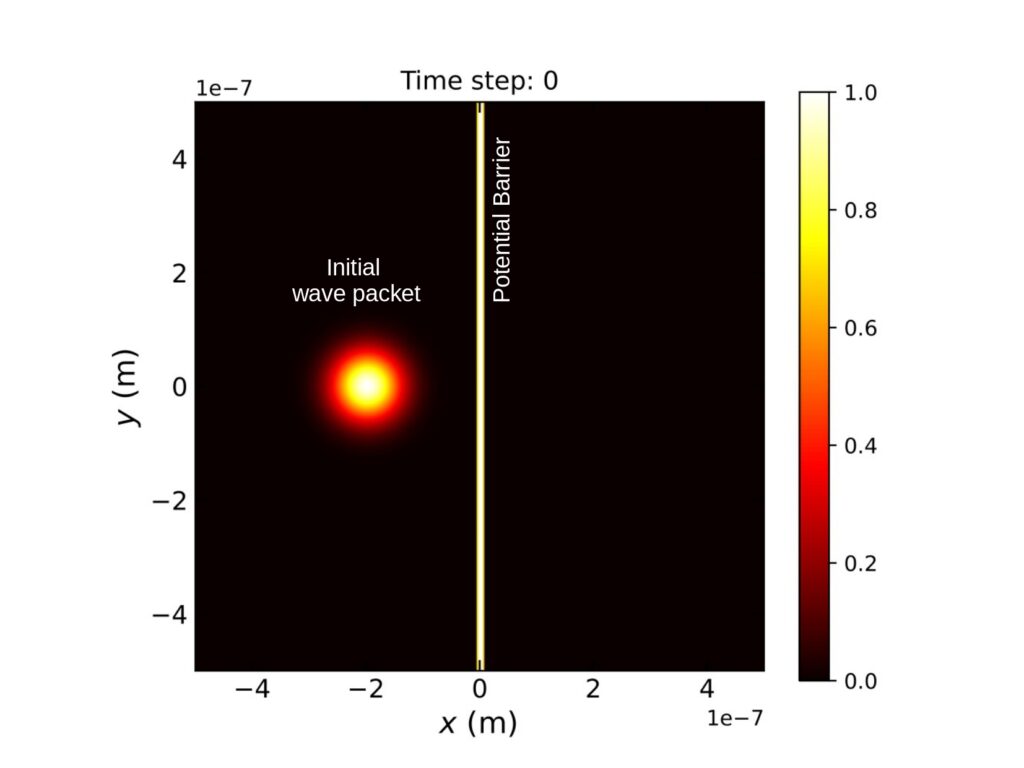
Setup of the Problem
Initial Wave Packet: Consider a 2D Gaussian wave packet with the form
\[\psi(x, y, t=0) = \frac{1}{\sqrt{2\pi\sigma_x\sigma_y}} \exp\left[-\frac{(x-x_0)^2}{4\sigma_x^2} – \frac{(y-y_0)^2}{4\sigma_y^2} + ik_0x\right],\]
where \( \sigma_x = \sigma_y = 60 \times 10^{-9} \) meters are the initial spreads of the wave packet in the \( x \) and \( y \) directions, respectively, \( x_0 = -2 \times 10^{-7} \) meters and \( y_0 = 0 \) are the initial positions, and \( k_0 = \frac{2\pi}{\lambda_{db}} \) with the de Broglie wavelength \( \lambda_{db} = 20 \times 10^{-9} \) meters is the initial wave vector in the \( x \)-direction.
Potential Barrier: The potential barrier is defined as,
\[V(x, y) = \begin{cases} 0 & \text{for } |x| > \frac{w}{2} \\V_0 & \text{for } |x| \leq \frac{w}{2}\end{cases},\]
where \( V_0 = 1.05 \times \frac{\hbar^2 k_0^2}{2 m_e} \) is the height of the barrier, and \( w = 10 \times 10^{-9} \) meters is the width of the barrier.
Time-Dependent Schrödinger Equation in 2D
The TDSE in 2D is given by,
\[i\hbar \frac{\partial \psi(x, y, t)}{\partial t} = -\frac{\hbar^2}{2m_e} \left(\frac{\partial^2 \psi(x, y, t)}{\partial x^2} + \frac{\partial^2 \psi(x, y, t)}{\partial y^2}\right) + V(x, y) \psi(x, y, t)\]
We can solve this equation numerically using methods like the Split-Step Fourier Method (SSFM) or the Finite Difference Time Domain (FDTD) method.
Numerical Implementation
Discretization
- Discretize the spatial domain into a grid with points \( x_i \) and \( y_j \), and discretize time into steps \( t_n \).
- Let \( \psi_{i,j}^n \) represent the wavefunction at grid point \( (x_i, y_j) \) and time \( t_n \).
Time Evolution Using Split-Step Fourier Method (SSFM)
The TDSE can be split into kinetic and potential evolution operators,
\[\psi(x, y, t + \Delta t) = e^{-iV(x,y)\Delta t/2\hbar} e^{-iH_k \Delta t/\hbar} e^{-iV(x,y)\Delta t/2\hbar} \psi(x, y, t),\]
where \( H_k = -\frac{\hbar^2}{2m_e}\nabla^2 \) is the kinetic energy operator.
- Step 1: Apply the potential evolution in real space
\[\psi(x_i, y_j, t + \Delta t/2) = \exp\left[-\frac{iV(x_i, y_j)\Delta t}{2\hbar}\right] \psi(x_i, y_j, t)\]
- Step 2: Apply the kinetic evolution in Fourier space. Transform \( \psi(x, y) \) to \( \tilde{\psi}(k_x, k_y) \) using a 2D Fourier transform
\[\tilde{\psi}(k_x, k_y, t + \Delta t) = \exp\left[-\frac{i\hbar(k_x^2 + k_y^2)\Delta t}{2m_e}\right] \tilde{\psi}(k_x, k_y, t)\]
Transform back to real space.
- Step 3: Apply the potential evolution again in real space
\[\psi(x_i, y_j, t + \Delta t) = \exp\left[-\frac{iV(x_i, y_j)\Delta t}{2\hbar}\right] \psi(x_i, y_j, t + \Delta t/2)\]
Calculating Reflection and Tunneling Probabilities
After evolving the wave packet over time, the reflection and tunneling probabilities can be calculated by integrating the probability density \( |\psi(x, y, t)|^2 \) over the relevant regions.
Reflection Probability \( R \):
\[R(t) = \int_{-\infty}^{0} \int_{-\infty}^{\infty} |\psi(x, y, t)|^2 dx dy\]
Tunneling Probability \( T \):
\[T(t) = \int_{\frac{w}{2}}^{\infty} \int_{-\infty}^{\infty} |\psi(x, y, t)|^2 dx dy\]
These integrals give the total probability of the wave packet being found in the regions \( x < 0 \) (reflection) and \( x > \frac{w}{2} \) (tunneling) at time \( t \).
| Parameter | Symbol/Variable | Value |
|---|---|---|
| Reduced Planck constant | \( \hbar \) | \( 1.0545718 \times 10^{-34} \) J·s |
| Electron mass | \( m_e \) | \( 9.10938356 \times 10^{-31} \) kg |
| de Broglie wavelength | \( \lambda_{\text{db}} \) | \( 20 \times 10^{-9} \) meters |
| Wave number | \( k_0 \) | \( \frac{2\pi}{\lambda_{\text{db}}} \) |
| Simulation domain size | \( L_x, L_y \) | \( 1 \times 10^{-6} \) meters |
| Number of grid points | \( N_x, N_y \) | 512 |
| Grid spacing | \( \Delta x, \Delta y \) | \( 1.9531 \times 10^{-9} \) meters |
| Time step | \( \Delta t \) | \( 3 \times 10^{-14} \) seconds |
| Number of time steps | \( N_t \) | 501 |
| Initial wave packet width | \( \sigma_x, \sigma_y \) | \( 60 \times 10^{-9} \) meters |
| Initial wave packet position | \( x_0, y_0 \) | \(-2 \times 10^{-7}\) meters, 0 meters |
| Initial wave vector | \( k_{x0}, k_{y0} \) | \( k_0, 0 \) |
| Barrier width | \( w \) | \( 1 \times 10^{-8} \) meters |
| Barrier height | \( V_0 \) | \( 1.05 \times \frac{\hbar^2 k_0^2}{2 m_e} \) |
| Fourier space components | \( k_x, k_y \) | – |
Quantum Tunneling Simulation Code in Python
import numpy as np
import matplotlib.pyplot as plt
# Constants
hbar = 1.0545718e-34 # Reduced Planck constant (Joule second)
m_e = 9.10938356e-31 # Mass of electron (kg)
wavelength = 20e-9 # de Broglie wavelength (meters)
k0 = 2 * np.pi / wavelength # Wave number
# Grid parameters
Lx, Ly = 1e-6, 1e-6 # Simulation domain size (meters)
Nx, Ny = 512, 512 # Number of grid points
dx, dy = Lx / Nx, Ly / Ny # Grid spacing
x = np.linspace(-Lx / 2, Lx / 2, Nx)
y = np.linspace(-Ly / 2, Ly / 2, Ny)
X, Y = np.meshgrid(x, y)
# Time parameters
dt = 3e-14 # Time step (seconds)
Nt = 501 # Number of time steps
# Wave packet parameters
sigma_x = sigma_y = 60e-9 # Width of the Gaussian packet (meters)
x0, y0 = -2e-7, 0 # Initial position of the packet (meters)
kx0, ky0 = k0, 0 # Initial wave vector
# Barrier parameters
barrier_width = 1e-8 # Width of the square barrier (meters)
barrier_height = 1.05 * hbar**2 * k0**2 / (2 * m_e) # Height of the barrier (Joules)
# Initial Gaussian wave packet
psi0 = np.exp(-(X - x0) ** 2 / (2 * sigma_x ** 2)) * np.exp(-(Y - y0) ** 2 / (2 * sigma_y ** 2))
psi0 = psi0.astype(np.complex128)
psi0 *= np.exp(1j * (kx0 * X + ky0 * Y))
# Normalize the initial wave packet
psi0 /= np.sqrt(np.sum(np.abs(psi0) ** 2))
# Potential energy (Square barrier in the center)
V = np.zeros_like(X)
V[np.abs(X) < barrier_width / 2] = barrier_height
# Fourier space components
kx = np.fft.fftfreq(Nx, dx) * 2 * np.pi
ky = np.fft.fftfreq(Ny, dy) * 2 * np.pi
KX, KY = np.meshgrid(kx, ky)
K2 = KX ** 2 + KY ** 2
# Split-step Fourier method
psi = psi0.copy()
transmission_prob = 0
for t in range(Nt):
# (a) 1/2 Evolution for the potential energy in real space
psi *= np.exp(-1j * V * dt / (2 * hbar))
# (b) Forward transform
psi_k = np.fft.fft2(psi)
# (c) Full evolution for the kinetic energy in Fourier space
psi_k *= np.exp(-1j * hbar * K2 * dt / (2 * m_e))
# (d) Inverse Fourier transform
psi = np.fft.ifft2(psi_k)
# (e) 1/2 Evolution for the potential energy in real space
psi *= np.exp(-1j * V * dt / (2 * hbar))
# Calculate transmission probability after time step 300
if t >= 0:
Rho = np.abs(psi) ** 2
transmission_prob = np.sum(Rho[X > barrier_width / 2])
# Visualization of wave packet evolution
if t % 50 == 0:
plt.figure(figsize=(6, 5))
Rho = np.abs(psi) ** 2
plt.imshow(Rho/np.max(Rho) + V/np.max(V), extent=(-Lx / 2, Lx / 2, -Ly / 2, Ly / 2), cmap='hot')
plt.colorbar()
plt.title(f'Time step: {t}\nTransmission Probability: {transmission_prob:.3f}')
plt.xlabel(r'$x$ (m)', fontsize=14)
plt.ylabel(r'$y$ (m)', fontsize=14)
plt.tick_params(which="major", axis="both", direction="in", top=True, right=True, length=5, width=1, labelsize=12)
plt.pause(1) # Pause for 1 second
plt.close()
Code Overview:
Here are the main steps in the code –
- Initialization: Define constants such as the reduced Planck constant, electron mass, and de Broglie wavelength. Set up the grid parameters, time steps, and initial wave packet characteristics.
- Potential Barrier Setup: Create a potential energy barrier in the center of the simulation domain with defined height and width.
- Initial Wave Packet Creation: Initialize a 2D Gaussian wave packet with a specified position, width, and wave vector.
- Normalization: Normalize the wave packet to ensure the total probability equals one.
- Fourier Space Components: Compute the Fourier space components for kinetic evolution.
- Time Evolution: Use the split-step Fourier method to evolve the wave packet in time, alternating between real and Fourier space operations.
- Transmission Probability Calculation: After a specified time step, calculate the transmission probability by integrating the probability density beyond the barrier.
- Visualization: Plot the wave packet evolution and the final state, including the transmission probability in the legend.
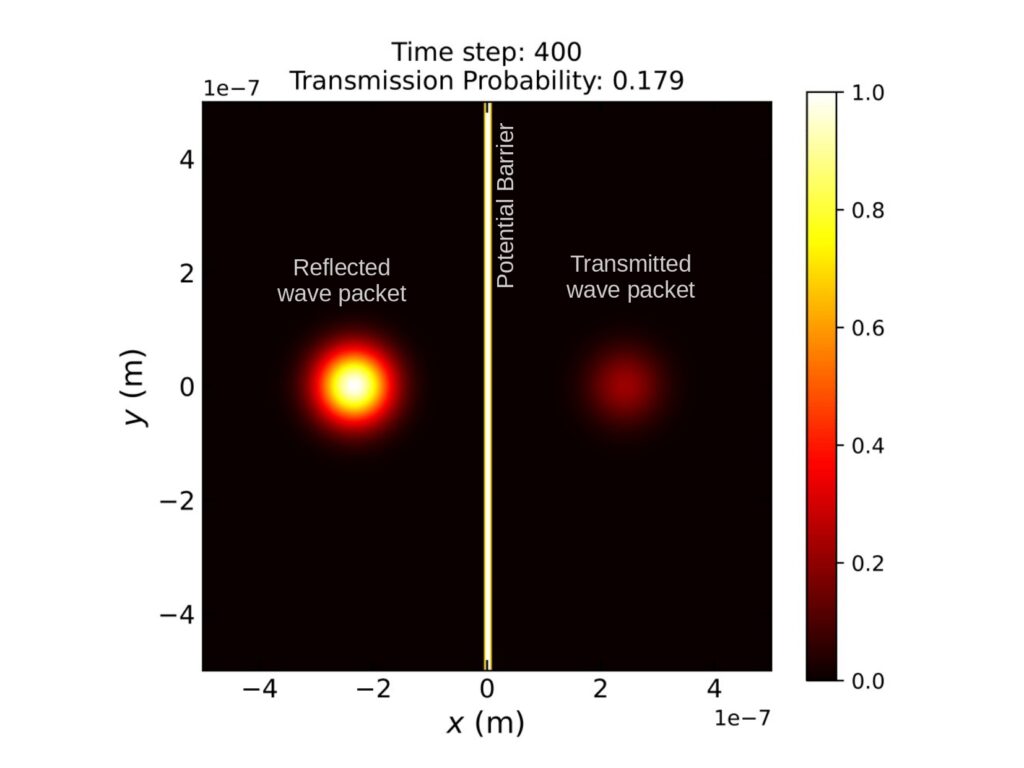
Simulation Results and Discussions
The simulation results illustrate the evolution of a 2D Gaussian wave packet as it interacts with a potential barrier, effectively demonstrating quantum tunneling. The barrier has a height that is (1.05) times the kinetic energy of the particle. As the wave packet encounters the barrier, a portion of it successfully tunnels through, while the rest is reflected. The transmission probability is computed to quantify the fraction of the wave packet that penetrates the barrier. The final visualization clearly depicts the wave packet’s spatial distribution across the simulation domain, with the transmission probability prominently displayed. This provides valuable insight into the tunneling phenomenon and the subsequent behavior of the wave packet post-barrier interaction.
Conclusion
This article provides a comprehensive overview of quantum tunneling, emphasizing its fundamental role in various physical phenomena. By exploring the theoretical framework and numerical simulation of a 2D Gaussian wave packet interacting with a potential barrier, we demonstrated how quantum mechanics allows particles to cross barriers that would be insurmountable in classical physics. The simulation results, particularly the transmission probability, offer a clear visualization of this phenomenon, highlighting the non-zero likelihood of a particle tunneling through a barrier even when its energy is below the barrier height. The study also underscores the significance of quantum tunneling in fields such as nuclear physics, semiconductor devices, and quantum computing, where it drives critical processes.
References
[1] Max, “Animation of Quantum Tunneling: A code in Python“, MatterWaveX.Com, August 13, (2024).

I am a science enthusiast and writer, specializing in matter-wave optics and related technologies. My goal is to promote awareness and understanding of these advanced fields among students and the general public.

